Question

A man wants to cut down a tree in his yard. To ensure that the tree doesn't hit anything, he needs to know the height of the tree. He measures his distance from the tree at 10 meters and angle of elevation to the tree at 54 degrees. What is the height of the tree to the nearest tenth of a meter?

Answer 1

the height of the tree to the nearest tenth of a meter is 13.8 meters

Explanation:

The computation of the height of the tree is shown below:

Given that

Distance from the tree is 10 meters

And, the angle of elevation of the tree is 54 degrees

Now here we used the trigonometry

`tan \theta = (Height)/(distance) \\\\tan 54^(\circ) = (height)/(10)`

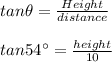
So, the height is

= 10 × 1.38

= 13.8 meters

hence, the height of the tree to the nearest tenth of a meter is 13.8 meters