Question

A go cart designer wants to build a Go Cart that measures 4 feet in height, 6 feet in width and 8 feet in length. He needs to construct a three - dimensional model of the Go Cart. Which measurements are appropriate for the scale model? a. 2 feet wide, 5 feet long and 2 feet high b. 3 feet wide, 4 feet long and 2 feet high c. 2.5 feet wide, 2 feet long, and 1.5 feet high d. 6 feet wide, 5 feet long and 3 feet high

Answer 1

The correct answer is:

3 feet wide, 4 feet long, and 2 feet high (b)

Explanation:

The appropriate scale for the model must be equivalent to the scale of the actual design. This means that the variation must be of the same magnitude in the length, height, and width.

Cart design = 4 feet high; 6 feet wide; 8 feet long

Cart design = 4H : 6W : 8L

where H, W, and L are height, width, and length respectively.

since the model of the Go Cart will be lesser than the actual Cart, let us divide the original cart design by the model designs given in the option, anyone that gives us a consistent variation is a right scale. This is done as follows:

a) Model design = 2H : 2W : 5L

dividing the original dimension by the model:

`(4H)/(2H) : (6W)/(2W) : (8L)/(5L) \\= 2 : 3 : 1.6\\`

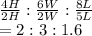
since the variations are not the same, this is the wrong model.

b) Model design = 2H : 3W : 4L

`(4H)/(2H) : (6W)/(3W) : (8L)/(4L) \\= 2 : 2 : 2\\`

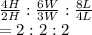
variation is the same across the three dimensions, hence this is the right model.

You can go ahead to use the same principle to do the third model, to see what you will get.