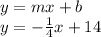Question

Y=4x-6 and passes through point 8,12

Answer 1

Slope of required line if both lines are parallel: y=4x-20

Slope of required line if both lines are perpendicular: y=-1/4x+14

Explanation:

We need to find equation of line while we are given another line having equation y=4x-6 and passes through point (8,12)

Note: Since it is not given if the required line is parallel or perpendicular to the given line. I will solve for both cases.

If Both lines are parallel the equation of new line will be:

We need to find slope and y-intercept to write equation of new line.

When lines are parallel there slopes are same

So, slope of given line y=4x-6 is 4 (Compare with general equation y=mx+b, m is slope so m=4)

Slope of new line is: m=4

Now finding y-intercept

Using slope m=4 and point (8,12) we can find y-intercept

`y=mx+b\\12=4(8)+b\\12=32+b\\b=12-32\\b=-20`

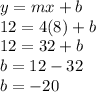
y-intercept of new line is: b=-20

Equation of required line having slope m=4 and y-intercept b=-20 is

`y=mx+b\\y=4x-20`

If Both lines are perpendicular the equation of new line will be:

We need to find slope and y-intercept to write equation of new line.

When lines are perpendicular there slopes are opposite of each other

So, slope of given line y=4x-6 is 4 (Compare with general equation y=mx+b, m is slope so m=4)

Slope of new line is: m=-1/4

Now finding y-intercept

Using slope m=-1/4 and point (8,12) we can find y-intercept

`y=mx+b\\12=-(1)/(4) (8)+b\\12=-2+b\\b=12+2\\b=14`

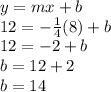
y-intercept of new line is: b=14

Equation of required line having slope m=-1/4 and y-intercept b=14 is

`y=mx+b\\y=-(1)/(4) x+14`