Answer:
The values of x and y are x = -3 and y = -2
Explanation:
In the complex numbers if a + bi = c + di, then the real parts are equal and the imaginary parts are equal
Let us use this rule to solve the question
∵ -21 + 5yi = 7x - 10i
∴ The real parts are -21 and 7x
∴ The imaginary parts are 5y and -10
∵ The real parts are equal
∴ 7x = -21
→ Divide both sides by 7
∴
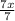 =
=
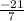
∴ x = -3
∵ The imaginary parts are equal
∴ 5y = -10
→ Divide both sides by 5
∴
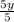 =
=
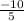
∴ y = -2
∴ The values of x and y are x = -3 and y = -2