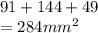Answer:
284 mm^2
Explanation:
We observe that the figure is made up of 2 squares and 1 triangle.
Lets consider the bigger square.
We observe that it sides are 12 mm
Hence, its area would be the square of its sides and hence, 144 mm^2.
Lets now consider the smaller square.
We observe that it sides are 7 mm
Hence, its area would be the square of its sides and hence, 49 mm^2.
Now,
Lets take the triangle into consideration.
We observe that,
One of its segments is not given to us and needs to be computed;
Lets take the length of this segment as x
Hence,
As the one of the sides of both the squares sum up to the lengths of the segment and 8,
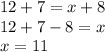
We now find its:
Base = 3+11 =14 mm
Height = 13mm
Hence, its area would be :
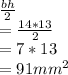
Hence,
The Sum of the total figure = Sum of Areas of both the squares and that of the triangle
Hence,
The Sum of the total figure =