Question

Does anyone has Algebra ll and if so can you please help me with this problem 5i/-2-6i And also I can't pay you but I hope God can give more good luck

Answer 1

`s = (5i)/(-2-6i) = -(30)/(40)-(10)/(40)i`

Explanation:

We have the following complex number
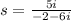`s = (5i)/(-2-6i)`, we proceed to simplify the expression as follows:

1)
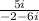`(5i)/(-2-6i)` Given.

2)
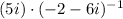`(5i)\cdot (-2-6i)^(-1)` Definition of division.

3)
`[(5i)\cdot (-2-6i)^(-1)]\cdot [(-2+6i)\cdot (-2+6i)^(-1)]` Modulative and associative properties/Existence of the additive inverser

4)
`[(5i)\cdot (-2+6i)]\cdot [(-2-6i)^(-1)\cdot (-2+6i)^(-1)]` Commutative and associative properties.

5)
`[(5i)\cdot (-2+6i)]\cdot [(-2-6i)\cdot (-2+6i)]^(-1)`
`a^(c)\cdot b^(c) = (a\cdot b)^(c)`

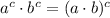
6)
`[(5i)\cdot (-2+6i)]\cdot [4+36]^(-1)`
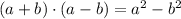`(a+b)\cdot (a-b) = a^(2)-b^(2)`/Definition of complex number/
`a\cdot (-b) = -a\cdot b`

7)
`[(5i)\cdot (-2)+(5i)\cdot (6i)]\cdot 40^(-1)` Definition of sum.

8)
`(-10\cdot i+30\cdot i^(2))\cdot 40^(-1)`
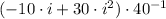
`a\cdot (-b) = -a\cdot b`/Associative and commutative properties.

9)
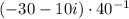`(-30-10i)\cdot 40^(-1)` Commutative properties/Definition of complex number/
`a\cdot (-b) = -a\cdot b`

10)
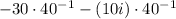`-30\cdot 40^(-1)-(10i)\cdot 40^(-1)` Distributive property.

11)
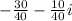`-(30)/(40)-(10)/(40)i` Definition of division/Result.

`s = (5i)/(-2-6i) = -(30)/(40)-(10)/(40)i`