Question

About how much greater is the gravitational force exerted by the moon on the near side of the earth than on the far side? Choose one of the Following:

(a) About 2% greater
(b) About 7% greater
(c) About 11% greater
(d) About 15% greater

Answer 1

Distance between the Earth and the moon, D =
`$3.84 * 10^8 \ m$`

Radius of the earth, r =
`$6.37 * 10^6 \ m$`

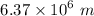
Gravitational force on the near side of the moon,
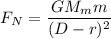`$F_N = (GM_m m)/((D-r)^2)$` ......(1)

Gravitational force on the far side of the moon,
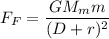`$F_F = (GM_m m)/((D+r)^2)$` ..............(2)

where,
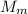`$M_m$` = mass of moon

m = mass of the near side and far side respectively (both are taken as equal)

Therefore,

`$(F_N-F_F)/(F_N)* 100 = ((GM_m m)/(D-r)^2-(GM_m m)/(D+r)^2)/((GM_m m)/(D-r)^2) * 100 $`

`$=1 - ((D-r)^2)/((D+r)^2) * 100$`

`$=1- \left((1-(r)/(D))/(1+(r)/(D))\right)^2 * 100$`

`$= 1- \left((0.98)/(1.02)\right)^2 * 100$`

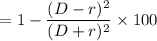
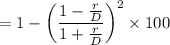
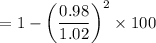
= 7%

Hence, correct option is (b) About 7% greater