Question

The height of an object thrown straight up from a height 5 feet above the ground is given by the equation h(t) =-16t^2 +42t +5, where is the time in seconds after the object is thrown. Find the average speed of the object from 1.5 to 2.25 seconds.

Answer 1

The average speed of the object is 18.67 ft/s.

Explanation:

Given;

height function, f(t) = -16t² + 42t + 5

The average speed is given by ;

`v = (dx)/(dt) \\\\h(t) = -16t^2+42t+5\\\\h(1.5)= -16(1.5)^2 + 42(1.5)+5 = 32 \ ft\\\\`

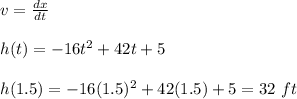
from 1.5 s to 2.25 s = 0.75 s,

`h(0.75)= -16(0.75)^2 + 42(0.75)+5 = 27.5 \ ft`

`h(2.25)= 27.5 +(-16(2.25)^2 + 42(2.25)+5) \\\\h(2.25) = 27.5 + 18.5 = 46 \ ft`

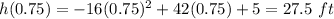
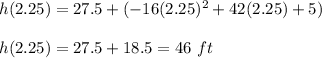
The average speed is given by ;

`v = (h(2.25) - h(1.5))/(2.25-1.5)\\\\v = (46-32)/(0.75)\\\\v = 18.67 \ ft/s`

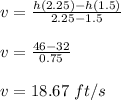
Therefore, the average speed of the object is 18.67 ft/s.