Question

Better Business Bureau conducted survey of 3,765 randomly selected residents of Laradise and found that 60% of them feel the businesses in Laradise, are run by compliant individuals. Find the best point estimate of the proportion of all residents of Laradise who believe businesses are run by compliant individuals.

a. In the prompt above what population parameter are you being asked about?

i. Proportion
ii. Mean
iii. Standard Deviation/Variance
iv. None of the above

b. What is the best point estimate?
c. What is the critical value (zα/2) or (tα/2) that corresponds to a confidence level of 99%?
d. Find the margin of error E, show the formula, fill in the pieces.
e. Construct the 99% confidence interval.

Answer 1

a

i. Proportion

b

Best point estimate is
`\^ p = 0.60`

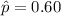
c

`z_{(\alpha )/(2) } =  2.58`

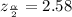
d

`E =  0.0206`

e

`0.5794 <  p < 0.6206`

Explanation:

From the question we are told that

The sample size is n = 3765

The proportion that feel the businesses in Laradise, are run by compliant individuals is
`\^ p = 0.60`

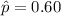
Considering question a

The correct option is Proportion because in the prompt we are told to obtain the fraction of the total sample size that has a particular attribute (which their opinion of business in Laradise) and this is what a proportion represents

Considering question b

The best point estimate is
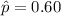`\^ p = 0.60` because this proportion best defines the fraction of the sample size who feel the businesses in Laradise, are run by compliant individuals

Considering question c

Generally given that the sample size is large enough n > 30 , it then means that the distribution is approximately normal so

From the question we are told the confidence level is 99% , hence the level of significance is

`\alpha = (100 - 99 ) \%`

=>
`\alpha = 0.01`

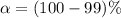
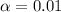
Generally from the normal distribution table the critical value of
`(\alpha )/(2)` is

`z_{(\alpha )/(2) } =  2.58`

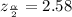
Considering question d

Generally the margin of error is mathematically represented as

`E =  Z_{(\alpha )/(2) } * \sqrt{(\^ p (1- \^ p))/(n) }`

=>
`E =  2.58 * \sqrt{( 0.60  (1- 0.60 ))/( 3765) }`

=>
`E =  0.0206`

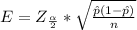
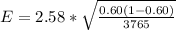
Considering question e

Generally 99% confidence interval is mathematically represented as

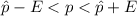
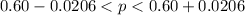
`\^ p -E <  p <  \^ p +E`

=>
`0.60  -0.0206 <  p < 0.60  + 0.0206`

=>
`0.5794 <  p < 0.6206`