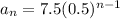Answer:
Number Sequence: a set of numbers that follow a pattern or a rule, where each number in the sequence is called a term.
Arithmetic Sequence: has a constant difference between each term, so the difference between each term is the same.
Geometric Sequence: has a constant ratio (multiplier) between each term, so each term is multiplied by the same number.
To determine the type of sequence, calculate the differences between the terms:
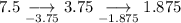
Therefore, this is not an arithmetic sequence, as the difference between the terms is not the same.
General form of a geometric sequence:
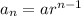
(where a is the first term and r is the common ratio)
To find the common ratio r, divide consecutive terms:

Therefore:
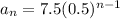
Finding the 4th and 5th terms:
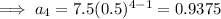
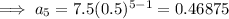
Part (a)

Part (b)
Geometric sequence
Part (c)