Question

A large room contains moist air at 30oC, 102 kPa. The partial pressure of water is 1.5 kPa. Determine(a) the relative humidity(b) the humidity ratio, in kg(vapor) per kg(dry air)(c) the dew point temperature, in oC(d) the mass of dry air, in kg if the mass of water vapor is 10 kg

Answer 1

Given :

Dry bulb temperature, T = 30 degree C

Absolute pressure, P = 102 kPa

Partial pressure of water vapor,
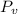`$P_v$` = 1.5 kPa

a). Relative humidity is given by

`$\phi = (P_v)/(P_(sat))$`

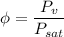
At T = 30 degree C, from the steam table, we have saturation temperature as 4.2469 kPa.

`$\phi = (1.5)/(4.2469)$`

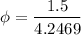
= 0.35319

= 35.31 %

b). Humidity ratio,

`$w= 0.622 * (P_v)/(P-P_v)$`

`$w= 0.622 * (1.5)/(102-1.5)$`

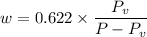
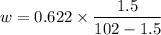
w = 0.009283 kg per kg of dry air

c). Dew point temperature is the saturation temperature at vapor pressure of air (can be found from steam table)

At
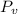`$P_v$` = 1.5 kPa, saturation temperature = 1.02 degree C

∴ Dew point temperature = 13.02 °C

d). Humidity ratio can be be defined as the ratio of mass of water vapor to the mass of dry air.

i.e.
`$w=(m_v)/(m_a)$`

`$0.009283 =(10)/(m_a)$`

∴
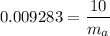
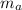`m_(a)` = 1077.170 kg of dry air