Question

Peter works as a delivery person for a bike shipping company. The graph shows a linear model for his

delivery times on different days.

(a) what is the equation of the line, first written in point-slope form and then written in slope- intercept form? show how you determined the equation.

(b) based on the linear model, predict how long it initially took peter to deliver his packages (y-intercept) approximately how much did his delivery time decrease per day (slope)? Complete sentences

Answer 1

Answer/Step-by-step explanation:

a. Using a point on the graph, (6, 12), and the slope of the line, we can first generate an equation in the point-slope form, given as
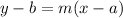`y - b = m(x - a)`, where,

m = slope, and (a, b) is a point on the line.

Using two points, (6, 12) and (3, 21), let's find slope, m.

`slope (m) = (y_2 - y_1)/(x_2 - x_1) = (21 - 12)/(3 - 6) = (9)/(-3) = -3`

Using a point (6, 12) and slope, m = -3, generate an equation in the point-slope form by substituting a = 6, b = 12, and m = -3 in
`y - b = m(x - a)`.

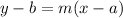✅Equation in point-slope form would be:

`y - 12 = -3(x - 6)`

Rewrite this to make it be in the slope-intercept form,
`y = mx + b`.

`y - 12 = -3(x - 6)`

`y - 12 = -3x + 18`

Add 12 to both sides

`y = -3x + 18 + 12`

`y = -3x + 30`

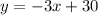
✅The equation in slope-intercept form is
`y = -3x + 30`

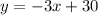
b. ✍️Based on the linear model,
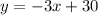`y = -3x + 30`, the 30 represents b = y-intercept.

✅Therefore, it took Peter 30 mins long initially to deliver his package.

✍️Based on the linear model,
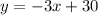`y = -3x + 30`, "-3" represents the slope.

✅Therefore, Peter's delivery time decreased 3 mins per day.