Question

What’s the answer to this question if your right I’ll keep giving you points

Answer 1

`\huge\text{$m\angle O=\boxed{11^(\circ)}$}`

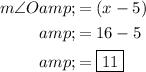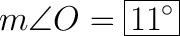
Since we know that all angles in a triangle add up to
`180^(\circ)`, we can solve for
`x` and substitute it back into
`(x-5)^(\circ)` to find
`m\angle O`.

`\begin{aligned}m\angle N+m\angle O+m\angle P&=180\\(5x-8)+(x-5)+(6x+1)&=180\end{aligned}`

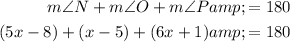
Remove the parentheses and combine like terms.

`\begin{aligned}5x-8+x-5+6x+1&=180\\(5x+x+6x)+(-8-5+1)&=180\\12x-12&=180\end{aligned}`

Add
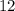`12` to both sides of the equation.

`\begin{aligned}12x-12&=180\\12x&=192\end{aligned}`

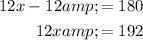
Divide both sides of the equation by
`12`.

`\begin{aligned}x=16\end{aligned}`

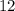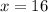
Now that we have the value of
`x`, we can substitute it back into
`(x-5)^(\circ)` to find
`m\angle O`.

`\begin{aligned}m\angle O&=(x-5)\\&=16-5\\&=\boxed{11}\end{aligned}`