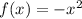Question

Which function has a vertex at the origin?

f(x) = (x + 4)²
f(x) = x(x-4)
f(x) = -x²
f(x) = (x-4)(x+4)

Answer 1

`f(x)=-x^2`

Explanation:

Vertex form of a quadratic function:

`f(x)=a(x-h)^2+k`

where:

• (h, k) is the vertex
• a is some constant

If the vertex is at the origin, then (h, k) = (0, 0)

`\implies f(x)=a(x-0)^2+0`

`\implies f(x)=ax^2`

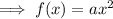
Comparing this with the answer options suggests that the function with a vertex at the origin is
`f(x)=-x^2`