Question

Given STU and DEF what is the m

Answer 1

m<E = 105°

Step-by-step explanation:

The ratio of the corresponding side lengths of ∆STU and ∆DEF are equal. That is:

`(ST)/(DE) = (TU)/(EF) = (SU)/(DF) = (15)/(6) = (10)/(4) = (20)/(8) = 2.5`.

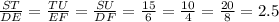According to similarly theorem, if two ∆s are similar, the ratio of their corresponding lengths would be the same. Therefore, ∆STU is similar to ∆DEF.

Since ∆STU ~ ∆DEF, their corresponding angles are congruent. That is:

<S ≅ <D, this means both are 29° each.

<T ≅ <E, this means they are both equal

<U ≅ <F. this means both are 46° each.

Thus:

m<E = 180 - (m<D + m<F) (sum of ∆)

m<E = 180 - (29 + 46) (substitution)

m<E = 180 - 75

m<E = 105°