Given:
Consider the graph passes through the point (5, −2) and has a slope of
 .
.
To find:
The equation of graph in standard form.
Solution:
Point slope form of a line is

where, m is slope and
 is the point lies on the line.
is the point lies on the line.
Slope is
 and graph passes through (5,-2), so the equation of line is
and graph passes through (5,-2), so the equation of line is
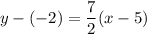
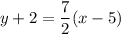
Multiply both sides by 2.

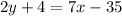
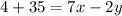

The required equation is
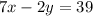 .
.
Therefore, the correct option is B.