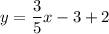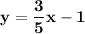Question

Whats the slope intercept form for the points [5,2] and [0,-1]

Answer 1

`\displaystyle y=(3)/(5)x-1`

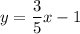
Explanation:

The Equation of a Line

The equation of the line in slope-intercept form is:

`y=mx+b`

Where m is the slope and b the y-intercept.

The equation of a line passing through points (x1,y1) and (x2,y2) can be found as follows:

`\displaystyle y-y_1=(y_2-y_1)/(x_2-x_1)(x-x_1)`

We are given the points (5,2) and (0,-1), thus:

`\displaystyle y-2=(-1-2)/(0-5)(x-5)`

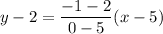
Operating:

`\displaystyle y-2=(-3)/(-5)(x-5)`

`\displaystyle y-2=(3)/(5)(x-5)`

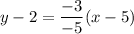
To find the slope-intercept form, we continue to simplify the expression:

Removing the parentheses:

`\displaystyle y-2=(3)/(5)x-(3)/(5)\cdot 5`

`\displaystyle y-2=(3)/(5)x-3`

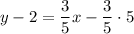
Adding 2:

`\displaystyle y=(3)/(5)x-3+2`

`\mathbf{\displaystyle y=(3)/(5)x-1}`