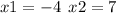Answer:
x¹= -4 , x²= 7
Explanation:
1) Determine the defined range
¼x-¾=7/x =/ 0
2) Move the expression to the left-hand side and change its sign
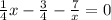
3) Write all numerators above the least common denominator 4x

4) When the quotient of expressions equals 0, the numerator has to be 0
x²-3x-28=0
5) Write -3x as a difference
x²+4x-7x-28=0
6) Factor out x from the expression
x(x+4)-7x-28=0
7) Factor out -7 from the expression
×(x+4)-7(x+4)=0
8) Factor out x+4 from the expression
(x+4)×(x-7)=0
9) When the product of the factors equals 0, at least one factor is 0
x+4=0
x-7=0
10) Solve for the x
x+4=0 -> x= -4
x-7=0 -> x=7
11) Check if the solution has defined range
x= -4
x=7
12) The solution has 2 solutions