Question

A 2.5. Gram arrow enters a 100 g apple with a speed of 115 m/s. If the apple was originally at rest, then what speed will it have as the arrow enters it? Remember the formula is P=MxV

Answer 1

The speed of the apple will be 2.81 m/s when the arrow enters it.

Step-by-step explanation:

We can find the speed of the apple by conservation of linear momentum:

`p_(i) = p_(f)`

`m_(ap)v_{ap_(i)} + m_(a)v_{a_(i)} = m_(ap)v_{ap_(f)} + m_(a)v_{a_(f)}`

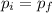
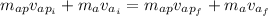
Where:

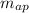`m_(ap)` is the mass of the apple = 100 g = 0.1 kg

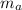`m_(a)` is the mass of the arrow = 2.5 g = 0.0025 kg

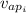`v_{ap_(i)}` and
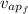`v_{ap_(f)}` is the initial and final speed of the apple respectively

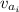`v_{a_(i)}` and
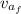`v_{a_(f)}` is the initial and final speed of the arrow respectively

Since the apple was originally at rest (
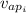`v_{ap_(i)}` = 0) and knowing that
`v_{a_(f)}` =
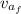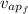`v_{ap_(f)}` when the arrow enters into the apple, we have:

`0 + 0.0025 kg*115 m/s = v(0.0025 kg + 0.1 kg)`

`v = 2.81 m/s`

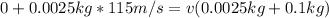
Therefore, the speed of the apple will be 2.81 m/s when the arrow enters it.

I hope it helps you!