Answer:
The two lines are neither
Explanation:
- Parallel lines have equal slopes and different y-intercepts
- Perpendicular lines have a product of their slopes = -1
- The slope of a line whose equation is ax + by = c is m =
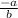
∵ The equations of the lines are
∵ In the 1st equation a = 8 and b = 1
∴ m1 =
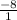 = -8
= -8
∴ The slope of the 1st line is -8
∵ In the 2nd equation a = 4 and b = 3
∴ m2 =
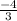
∴ The slope of the 2nd line is
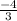
→ By using the facts above
∵ m1 ≠ m2
∴ The two lines are not parallel
∵ m1 × m2 = -8 ×
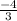 =
=
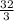
∴ m1 × m2 ≠ -1
∴ The two lines are not perpendicular
∴ The two lines are neither parallel nor perpendicular