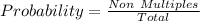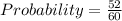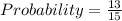Question

A bowl of raffle tickets contains the numbers 1 through 60. What is the probability that a randomly selected ticket will be a number that is not a multiple of 7

Answer 1

Explanation:

There are 8 numbers between 24 and 31, inclusive. So the probability of getting a number in that range is 885. Therefore, by the complement rule, the probability of not getting one of these numbers is

`1-(8/85)= 85- 8 /85=77/ 85`

Answer 2

`Probability = (13)/(15)`

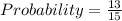
Explanation:

Given

Ticket Numbers = 1 to 60

Required

Determine the probability of not selecting multiples of 7

First, we need to list out the multiples of 7 in this range:

`Multiple = 7,14,21,28,35,42,49,56`

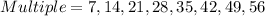
These are 8 in numbers. So, there are 8 multiples

Next, is that we determine the number of non multiples of 7. This s calculated as:

`Non\ Multiples = 60 - 8`

`Non\ Multiples = 52`

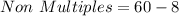
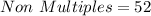
The required probability is then calculated as:

`Probability = (Non\ Multiples)/(Total)`

`Probability = (52)/(60)`

`Probability = (13)/(15)`