Answer:
C.
Explanation:
Transformations within the quadratic is given by the form:

Where a is the vertical stretch, h is the horizontal translations, and k is the vertical translations.
We have:
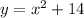
If we translate this 5 units to the right, we are letting h=5. This yields:
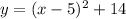
If we shift the function down 6 units, we are subtracting 6 from the function. This will yield:
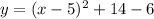
Subtract:
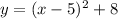
Therefore, our answer is C.