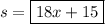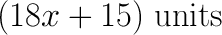
Given the area, we need to find the side length. Let's start with the area equation for a square, where
 is the side length and
is the side length and
 is the area.
is the area.
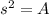
Substitute in the known value.
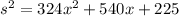
Now we need to factor the trinomial. The trinomial given for the area is a perfect square trinomial since
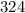 (
(
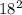 ) and
) and
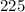 (
(
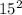 ) are both perfect squares.
) are both perfect squares.
We also know that
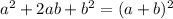 , which we can use to factor.
, which we can use to factor.
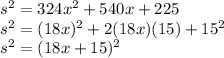
Now, take the square root of both sides to fully isolate
 .
.
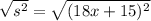
Keep in mind that the square root of any value squared is the original value.