Question

An object is swung in a horizontal circle on a length of string that is 0.93 m long. Its acceleration is 26.36 m/s2. What is the time it takes the object to complete one horizontal circle?

P.S. : I know the answer is 1.18 seconds but I just don’t know how to get there. Can someone explain to me how to do it? Thanks

Answer 1

The object takes approximately 1.180 seconds to complete one horizontal circle.

Step-by-step explanation:

From statement we know that the object is experimenting an Uniform Circular Motion, in which acceleration (
`a`), measured in meters per square second, is entirely centripetal and is expressed as:

`a = (4\pi^(2)\cdot R)/(T^(2))` (1)

Where:

`T` - Period of rotation, measured in seconds.

`R` - Radius of rotation, measured in meters.

If we know that
`a = 26.36\,(m)/(s^(2))` and
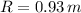`R = 0.93\,m`, then the time taken by the object to complete one revolution is:

`T^(2) = (4\pi^(2)\cdot R)/(a)`

`T = 2\pi\cdot \sqrt{(R)/(a) }`

`T = 2\pi\cdot \sqrt{(0.93\,m)/(26.36\,(m)/(s^(2)) ) }`

`T \approx 1.180\,s`

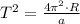
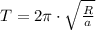
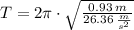
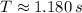
The object takes approximately 1.180 seconds to complete one horizontal circle.