Answer:
a
The null hypothesis is
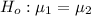
The alternative hypothesis
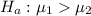
b
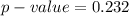
c
The decision rule is
Fail to reject the null hypothesis
Explanation:
From the question we are told that
The value given is
S/N
1 7 5
2 4 3
3 8 7
4 8 8
5 7 9
6 7 5
7 6 5
Generally the sample mean for the first sample is mathematically represented as
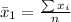
=>
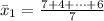
=>
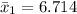
Generally the sample mean for the second sample is mathematically represented as
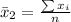
=>
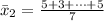
=>
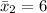
Generally the sample standard deviation for the first sample is mathematically represented as
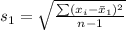
=>
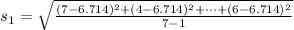
=>
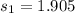
Generally the sample standard deviation for the second sample is mathematically represented as

=>

=>
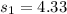
Generally the pooled standard deviation is
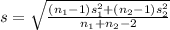
=>
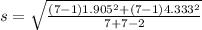
=>
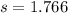
The null hypothesis is
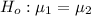
The alternative hypothesis
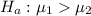
Generally the test statistics is mathematically represented as
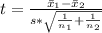
=>
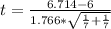
=>
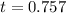
Generally the degree of freedom is mathematically represented as

=>

=>
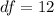
From the t distribution table the probability of
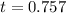 at a degree of freedom of
at a degree of freedom of
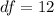 is
is
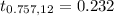
Generally the p-value is
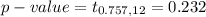
From the values obtained we see that
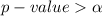 hence
hence
The decision rule is
Fail to reject the null hypothesis