Question

Find all points (x, y) where the functions f(x), g(x), h(x) have the same value: f(x) = 2^(x−5) + 3, g(x) = 2x − 5, h(x) = 8/x + 10

very fast please

Answer 1

The point where all three lines meet is (8, 11)

Explanation:

The given functions are;

f(x) = x²⁽ˣ ⁻ ⁵⁾ + 3

g(x) = 2·x - 5

h(x) = 8/x + 10

Equating the simpler functions g(x) to h(x) to find the points of the same value gives;

2·x - 5 = 8/x + 10

2·x - 15 = 8/x

2·x² - 15·x = 8

2·x² - 15·x - 8 = 0

With the aid of an online application, we have;

(2·x + 1)(x - 8) = 0

x = -1/2, or x = 8

The y-values at the point of intersection, is given as follows;

g(x) = 2·x - 5 = 2×(-1/2) - 5 = -6 or g(x) = 2·x - 5 = 2×(8) - 5 = 11

The points where g(x) and h(x) meet are (-1/2, -6) and (8, 11)

To check where the point of intersection of the two functions g(x) ang h(x) meet f(x), we have;

At x = -1/2, f(x) =
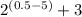`2^((0.5 - 5)) + 3` = 3.0442

At x = -1/2, f(x) = 2⁽⁸⁻⁵⁾ + 3 = 8 + 3 = 11

Therefore, the point where all three lines meet = (8, 11).