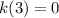Answer:

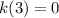
Explanation:
Given:
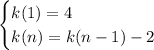
The function
 tells us that each term is 2 less than the preceding term.
tells us that each term is 2 less than the preceding term.
Therefore:
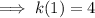

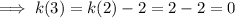
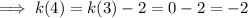
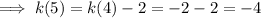
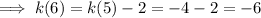
Rearranging the formula to make the preceding term the subject:
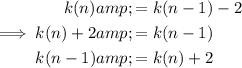
Therefore:
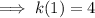
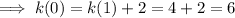
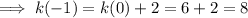
Conclusion
Therefore, the correct answers from the answer options are:
 and
and