Question

Need help asap please!

Answer 1

• determinant: -15
• x = 3; y = 4; z = 1

Explanation:

The matrix of coefficients has one row corresponding to each equation. The constants in that row are the coefficients of the variables in the equation. Coefficients are listed in the same order on each row. A missing term is represented by a coefficient of 0.

coefficient matrix, determinant

The first attachment shows the coefficient matrix and its determinant.

__

solution

The solution to the system of equations can be found by left-multiplying the constant vector by the inverse of the coefficient matrix.

`\textbf{A$\cdot$X}=\textbf{B}\\\\\textbf{X}=\textbf{A}^(-1)\cdot\textbf{B}`

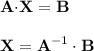
This multiplication is shown in the second attachment. It tells us ...

`\textbf{X}=\left[\begin{array}{c}x\\y\\z\end{array}\right]=\left[\begin{array}{c}3\\4\\1\end{array}\right]`