Question

If a rock is dropped from the top of a tower at the front of it and takes 3.6 seconds to hit the ground. Calculate the final velocity of the penny in m/s. What is the height of the tower?

Answer 1

35.28m/s; 63.50m

Step-by-step explanation:

Given the following data;

Time, t = 3.6 secs

Since it's a free fall, acceleration due to gravity = 9.8m/s²

Initial velocity, u = 0

To find the final velocity, we would use the first equation of motion;

`V = u + at`

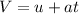
Substituting into the equation, we have;

`V = 0 + 9.8 * 3.6`

V = 35.28m/s

Therefore, the final velocity of the penny is 35.28m/s.

To find the height, we would use the second equation of motion;

`S = ut + \frac {1}{2}at^(2)`

Substituting the values into the equation;

`S = 0(3.6) + \frac {1}{2}*9.8*(3.6)^(2)`

`S = 0 + 4.9*12.86`

`S = 0.5 *36`

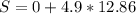
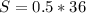
S = 63.50m

Therefore, the height of the tower is 63.50m.