Question

If the present value of an item is P

and we experience an inflation rate
of r for t years, what will the future
value of the item be?
P = $100
r = 4%
t = 15

Answer 1

inflation means, the same item costs more however is the same item, so if a tomato in January 1st costs $1 and by December 31st it costs $2, the price went up by twice, however is same tomato, it didn't become twice as large, anyhow, inflation eats away value and thus is a Decay case.

`\qquad \textit{Amount for Exponential Decay} \\\\ A=P(1 - r)^t\qquad \begin{cases} A=\textit{current amount}\\ P=\textit{initial amount}\dotfill &100\\ r=rate\to 4\%\to (4)/(100)\dotfill &0.04\\ t=\textit{elapsed time}\dotfill &15\\ \end{cases} \\\\\\ A=100(1 - 0.04)^(15)\implies A=100(0.96)^(15)\implies A\approx 54.21`

now, we can look at this value reduction as value that was eaten away from the original.

we can also see the inflationary effect as the new value of the tomato in money terms, that means the tomato went up in price, exponentially by 4% per year, so we can see that as a Growth case in terms of money spent on it.

`\qquad \textit{Amount for Exponential Growth} \\\\ A=P(1 + r)^t\qquad \begin{cases} A=\textit{accumulated amount}\\ P=\textit{initial amount}\dotfill &100\\ r=rate\to 4\%\to (4)/(100)\dotfill &0.04\\ t=\textit{elapsed time}\dotfill &15\\ \end{cases} \\\\\\ A = 100(1 + 0.04)^(15) \implies A \approx 180.09`

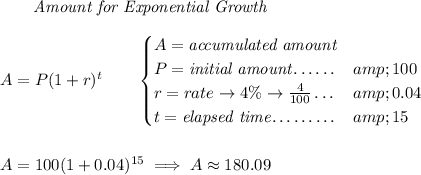
now, this is how much the tomato will be priced in money terms in 15 years.