Question

Let (-3, 5) be a point on the terminal side of 0.

Find the exact values of cos0, csc 0, and tan 0.

Answer 1

`\displaystyle \large{\cos \theta = (-3)/(√(34)) = -(3√(34))/(34)}\\\\\displaystyle \large{\csc \theta = (√(34))/(5)}\\\\\displaystyle \large{\tan \theta = -(5)/(3)}`

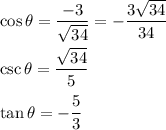
Explanation:

Accorded to trigonometric formula, we know that:

`\displaystyle \large{\cos \theta = (x)/(r)}\\\\\displaystyle \large{\csc \theta = (1)/(\sin \theta) = (1)/((y)/(r)) = (r)/(y)}\\\\\displaystyle \large{\tan \theta = (\sin \theta)/(\cos \theta) = (y)/(x)}`

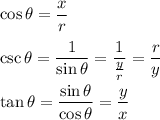
We know what x and y are but not yet knowing what r is. We can find r (radius) using the formula:

`\displaystyle \large{r=√(x^2+y^2)}`

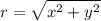
Input x = -3 and y = 5 in.

`\displaystyle \large{r=√((-3)^2+(5)^2)}\\\\\displaystyle \large{r=√(9+25)}\\\\\displaystyle \large{r=√(34)}`

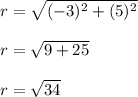
Hence, radius is √34 and we know all we need now. Substitute x = -3, y = 5 and r = √34 in respective areas.

`\displaystyle \large{\cos \theta = (-3)/(√(34)) = -(3√(34))/(34)}\\\\\displaystyle \large{\csc \theta = (√(34))/(5)}\\\\\displaystyle \large{\tan \theta = -(5)/(3)}`

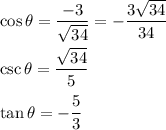
These are the answers to your question. Please let me know if you have any questions regarding my answer or explanation!