The factored form is (a + 5)(a - 5) - 2ab
The expression
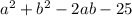 can be factored as a product of two binomials.
can be factored as a product of two binomials.
To factor it, we look for two numbers whose product is the product of the square of the first term and the square of the last term, and whose sum is the middle term.
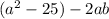
Now,
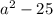 can be further factored as the difference of squares:
can be further factored as the difference of squares:
(a + 5)(a - 5) - 2ab
So, the factored form is (a + 5)(a - 5) - 2ab