Question

A box of mass 60 kg is at rest on a horizontal floor that has a static coefficient of friction of 0.6 and a kinetic coefficient of 0.25

a)The minimum force necessary to start moving the box
b) The friction force and the acceleration of the box if a horizontal force of 400 N is applied

Answer 1

a) F = 353.2 N

b)
`F_(f) = 147.2 N`

a = 4.21 m/s²

Step-by-step explanation:

a) The minimum force necessary to start moving the box s given by:

`\Sigma F = 0`

`F - \mu_(s)N = 0`

`F = \mu_(s)mg`

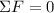
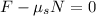
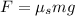
Where:

F: is the force applied to move the box

μs: is the static coefficient of friction = 0.6

m: is the mass = 60 kg

g: is the gravity = 9.81 m/s²

`F = 0.6*60 kg*9.81 m/s^(2) = 353.2 N`

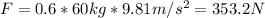
b) The acceleration is:

`F - F_(f) = ma`

`F - \mu_(k)mg = ma`

`a = (F - \mu_(k)mg)/(m) = (400 N - 0.25*60 kg*9.81 m/s^(2))/(60 kg) = 4.21 m/s^(2)`

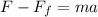
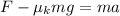
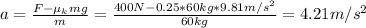
Now, the friction force is:

`F_(f) = \mu_(k)mg = 0.25*60 kg*9.81 m/s^(2) = 147.2 N`

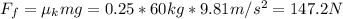
I hope it helps you!