Answer:
i. 1.84 m
ii. The ball's time in the air is 1.23s.
Step-by-step explanation:
The ball moves upward (against the gravitational pull) with an initial velocity of 6 m/s.
I. Applying the third equation of free fall, the maximum height is;
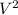 =
=
 - 2gs
- 2gs
where the variables have there usual meaning.
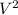 = 0 m/s,
= 0 m/s,
 = 6 m/s, g = 9.8 m/
= 6 m/s, g = 9.8 m/
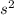 .
.
0 =
 - 2 x 9.8 x s
- 2 x 9.8 x s
0 = 36 - 19.6s
⇒ 19.6s = 36
s =
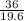
= 1.8367
= 1.84 m
The maximum height of the ball is 1.84 m.
II. The time the ball was in air can be determined by,
time of flight, T =
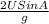
where U is the initial velocity of the ball, A is the angle of projection and g is the acceleration due to gravity.
U = 6 m/s, A =
 (since the ball is thrown vertically upward), and g = 9.8 m/
(since the ball is thrown vertically upward), and g = 9.8 m/
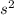 .
.
T =
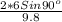
=
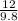
= 1.2245
T = 1.23s
The ball's time in the air is 1.23s.