Question

Decide whether quadrilateral ABCD with vertices 4(3,1), B(-1,-1) C(1,3), and D(5,5) is a rectangle, rhombus,

square, or parallelogram.

Answer 1

Answer: rectangle

Explanation:

From the options, it is implied that it is a parallelogram.

From inspection, we can tell that not all the sides are congruent, so it is not a rhombus or a square.

To determine if it is a rectangle, we can use the slope formula to see if there is a pair of perpendicular sides (if this is the case, then this will be a parallelogram with a right angle, making it a rectangle)

`m_{\overline{AB}}=(1-0)/(-4-(-3))=-1\\m_{\overline{BC}}=(4-1)/(-1-(-4))=1\\\therefore \overline{AB} \perp \overline{BC} \text{ because} \left(m_\overline{AB} \right) \left(m_{\overline{BC} \right)=1`

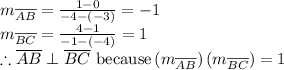
So, the most specific classification is a rectangle.