Answer:
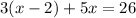 (Given Equation)
(Given Equation)
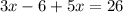 (Distributive Property)
(Distributive Property)
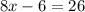 (Combine Like Terms)
(Combine Like Terms)
 (Addition Property of Equality)
(Addition Property of Equality)
 (Division Property of Equality)
(Division Property of Equality)
Explanation:
To justify the solution paths of linear equations.
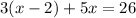 (It is the Given Equation)
(It is the Given Equation)
Here, 3 has to be multiplied with the term
 , so the distributive property applies.
, so the distributive property applies.
Therefore,
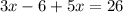
Here, the like terms with
 in it will have to solved by combining them into one.
in it will have to solved by combining them into one.
Therefore,
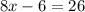
Here, we add 6 to both sides.

Now, divide the both sides with 8.

Therefore, the answer is:
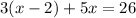 (Given Equation)
(Given Equation)
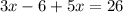 (Distributive Property)
(Distributive Property)
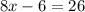 (Combine Like Terms)
(Combine Like Terms)
 (Addition Property of Equality)
(Addition Property of Equality)
 (Division Property of Equality)
(Division Property of Equality)