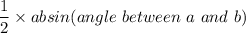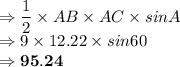Question

Given: △ABC, m∠A=60°

m∠C=45°, AB = 9
Find: Perimeter of △ABC
Area of △ABC
ABC is scalene

Answer 1

Perimeter = 32.3

Area = 95.24

Explanation:

Given:

△ABC, m∠A=60°

m∠C=45°, AB = 9

To find:

Perimeter of △ABC

Area of △ABC

Solution:

Using angle sum property in a triangle:

m∠A + m∠B + m∠C = 180°

m∠B = 180° - 45° - 60° = 75°

As per Sine Rule:

`(a)/(sinA) = (b)/(sinB) = (c)/(sinC)`

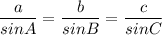
Where

`a` is the side opposite to
`\angle A`

`b` is the side opposite to
`\angle B`

`c` is the side opposite to
`\angle C`

`(BC)/(sin60^\circ) = (AC)/(sin 75^\circ) = (AB)/(sin 45^\circ) \\\Rightarrow (BC)/((\sqrt3)/(2)) = (9)/((1)/(\sqrt2)) \\\Rightarrow BC = 12.73* 0.87 \\\Rightarrow BC = 11.08`

`\Rightarrow (AC)/(0.96) = (9)/((1)/(\sqrt2)) \\\Rightarrow AC = 12.73* 0.96 \\\Rightarrow AC = 12.22`

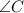
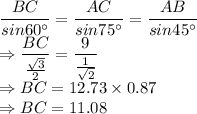
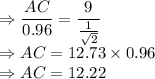
Perimeter of △ABC = AB + BC + AC = 9 + 11.08 + 12.22 = 32.3

Area of a triangle is given as:

`\frac{1}2* ab sin(angle\ between\ a\ and\ b)`

`\Rightarrow (1)/(2)* AB* AC* sinA\\\Rightarrow 9* 12.22* sin 60\\\Rightarrow \bold{95.24}`