Answer:
t = 2.38 [s]
Step-by-step explanation:
To solve this problem we must use the following equation of kinematics. We must clarify that both the acceleration and the initial velocity were taken as positive, since the velocity of the movement coincides with the direction of the acceleration.
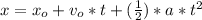
where:
x - Xo = distance = 47 [m]
Vo = initial velocity = 8 [m/s]
a = gravity acceleration = 9.81 [m/s²]
t = time [s]
Now replacing these values in the equation:
47 = 8*t + 0.5*9.81*t²
47 = 8t + 4.905t²
47 = 4.905*t(1.63 + t)
9.58 = t*(1.63 + t) solving this equation (cuadratic)
we found that t = 2.38 [s]