Answer:
y = 3x + 9
Explanation:
The perpendicular bisector to the line segment is passing through the midpoint of the line segment. Coordinates of midpoint are (
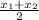 ,
,
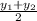 )
)
Formula of slope is m =
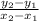
Slopes of perpendicular lines are opposite reciprocals. So, if AB ⊥ CD , then
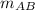 ×
×
 = - 1
= - 1
y -
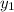 = m ( x -
= m ( x -
 )
)
~~~~~~~~~~~
(- 8, 5)
(4, 1)
m = ( 5 - 1) / ( - 8 - 4) = -

Opposite reciprocal to ( -
 ) is 3
) is 3
Coordinates of midpoint are ( - 2 , 3 )
y - 3 = 3 ( x + 2 )
y = 3x + 9