Question

ANSWER ASAP PLEASE AND SHOW YOUR WORK!!!!

Algebraically determine whether the following function is Even, Odd, or Neither.
f(x)= x^3-2x

Answer 1

f(-x)= -f(x) so, the given function
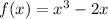`f(x)= x^3-2x` is Odd

Explanation:

We need to determine if the function
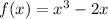`f(x)= x^3-2x` is Even, Odd, or Neither.

Determining if a function f(x) is even or not we put x=-x and check

If f(-x)=f(x) the function is even

if f(-x)= -f(x) the function is odd

So, Putting x =-x and determining if the given function
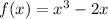`f(x)= x^3-2x` is Even, Odd, or Neither.

`f(x)= x^3-2x\\Put \ x=-x\\f(-x)=(-x)^3-2(-x)\\f(-x)=-x^3+2x\\f(-x)=-(x^3-2x)\\f(-x)=-f(x)`

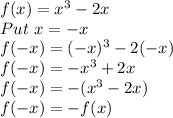
As f(-x)= -f(x) so, the given function
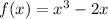`f(x)= x^3-2x` is Odd