Answer:
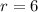
Explanation:
Make an equation in point-slope form where:
- the equation is
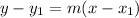
- y1 and x1 are the corresponding coordinate points
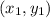
Insert the known values:
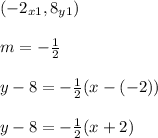
Use the distributive property:
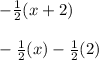
Simplify. Turn the 2 into a fraction by converting to the fraction
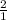 , which is still equal to 2:
, which is still equal to 2:
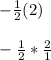
Multiply across:
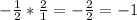
Re-insert into the equation:

Solve for y. Add 8 to both sides (to isolate y using inverse operations):
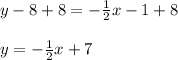
Now insert the y value of the other point to find r (which will have the same value as x):
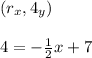
Simplify the fraction by multiplying. Add 1 as the denominator for x:
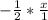
Multiply across:
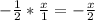
Re-insert:
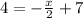
Subtract 7 from both sides (to isolate the variable using inverse operations):
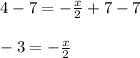
Multiply both sides by 2 (to undo the fraction using inverse operations):

Divide both sides by -1 (to make the variable positive, since two negatives make a positive):
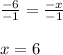
Therefore, the value of r is 6.
:Done