Question

In a control system, an accelerometer consists of a 4.63-g object sliding on a calibrated horizontal rail. A low-mass spring attaches the object to a flange at one end of the rail. Grease on the rail makes static friction negligible, but rapidly damps out vibrations of the sliding object. When subject to a steady acceleration of 0.832g, the object should be at a location 0.450 cm away from its equilibrium position.

Required:
Find the force constant of the spring required for the calibration to be correct.

Answer 1

8.4 N/m

Step-by-step explanation:

m = Mass of block = 4.63 gm

g = Acceleration due to gravity =
`9.81\ \text{m/s}^2`

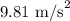
x = Displacement of spring = 0.45 cm

a = Acceleration of subject = 0.832g

k = Spring constant

Force is given by

`F=ma`

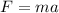
From Hooke's law

`F=kx`

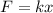
So

`ma=kx\\\Rightarrow k=(ma)/(x)\\\Rightarrow k=(4.63* 10^(-3)* 0.832* 9.81)/(0.45* 10^(-2))\\\Rightarrow k=8.4\ \text{N/m}`

The force constant of the spring is 8.4 N/m.