Question

A meat inspector has randomly selected 30 packs of 95% lean beef. The sample resulted in a mean of 96.2% with a sample standard deviation of 0.8%. Calculate an upper prediction bound for the leanness of a new pack using a prediction level of 99%. Assume normality. The contents of seven similar containers of sulfuric acid are 9.8, 10.2, 10.4, 9.8,10.0, 10.2, and 9.6 liters. Find a 95% confidence interval for the mean contents of all such containers, assuming an approximately normal distribution.

Answer 1

a

The upper bound of the 99% prediction level is
`98.2`

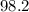
b

The 95% confidence interval is
`9.7383 <  \mu < 10.2617`

Explanation:

Considering first question

From the question we are told that

The sample size is n = 30

The sample mean is
`\= x = 96.2\%`

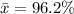
The standard deviation is
`s = 0.8\%`

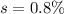
Generally the degree of freedom is mathematically represented as

`df = n - 1`

=>
`df = 30 - 1`

=>
`df = 29`

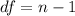
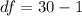
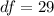
From the question we are told the confidence level is 99% , hence the level of significance is

`\alpha = (100 - 99 ) \%`

=>
`\alpha = 0.01`

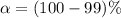
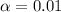
Generally from the t distribution table the critical value of at a degree of freedom of is

`t_(\alpha , 29) = 2.462`

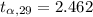
Generally the 99% prediction level is mathematically represented as

`\= x \pm [(t_(\alpha  , df )) * s * (\sqrt{1 + (1)/( n) } )}]`

Generally the upper bound of the 99% prediction level is mathematically represented as

`\= x + [(t_(\alpha  , df )) * s * (\sqrt{1 + (1)/( n) } )}]`

=>
`96.2 + (2.462 ) * 0.8 * (\sqrt{1 + (1)/( 30) } )}]`

=>
`98.2`

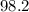
Considering second question

Generally the sample is mathematically represented as

`\= x = (\sum x_i)/(n)`

=>
`\= x = ( 9.8 + 10.2 + \cdots +9.6 )/(7)`

=>
`\= x = 10`

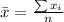
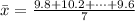
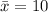
Generally the standard deviation is mathematically represented as

`\sigma = \sqrt{ ( \sum ( x_ i - \= x))/(n-1) }`

=>
`\sigma = \sqrt{ ( ( 9.8 -10)^2 + ( 10.2 -10)^2 + \cdots + ( 9.6 -10)^2 )/(7-1) }`

=>
`\sigma = 0.283`

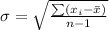
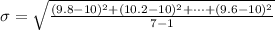
Generally the degree of freedom is mathematically represented as

`df =  n- 1`

=>
`df =  7- 1`

=>
`df =  6`

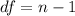
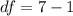
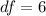
From the question we are told the confidence level is 95% , hence the level of significance is

`\alpha = (100 - 95 ) \%`

=>
`\alpha = 0.05`

Generally from the t distribution table the critical value of at a degree of freedom of is

`t_{(\alpha )/(2) , 6 } =  2.447`

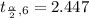
Generally the margin of error is mathematically represented as

`E = t_{(\alpha )/(2) , 6 } *  (\sigma )/(√(n) )`

=>
`E =2.447*    (0.283 )/(√(7) )`

=>
`E =0.2617`

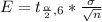
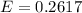
Generally 95% confidence interval is mathematically represented as

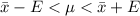
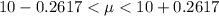
`\= x -E <  \mu <  \=x  +E`

=>
`10 -0.2617 <  \mu < 10 + 0.2617`

=>
`9.7383 <  \mu < 10.2617`