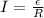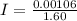Question

A flat loop of wire consisting of a single turn of cross-sectional area 7.10 cm2 is perpendicular to a magnetic field that increases uniformly in magnitude from 0.500 T to 2.10 T in 1.07 s. What is the resulting induced current if the loop has a resistance of 1.60?

Answer 1

The induced current is
`I = 0.00066 \ A`

Step-by-step explanation:

From the question we are told that

The area is
`A = 7.10 \ cm^2 = 7.10 *10^(-4) \ m^2`

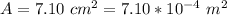
The initial magnetic field is
`B_i = 0.500 \ T`

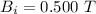
The magnetic field after t =1.07 s is
`B_f = 2.10 \ T`

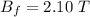
The resistance of the loop is
`R = 1.60 \ \Omega`

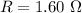
Generally the electromagnetic field induced is mathematically represented as

`\epsilon = NA * (B_f - B_i)/(t)`

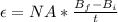
Where N is the number of turns which is 1 in the case of this question since there is only one loop

So

`\epsilon = 1 * 7.10*10^(-4)* (2.10 - 0.500)/(1.07 )`

=>
`\epsilon = 0.00106 \ V`

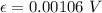
Generally the value of the current is mathematically represented as

`I = (\epsilon)/(R)`

`I = (0.00106)/(1.60)`

`I = 0.00066 \ A`