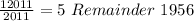Question

When a number n is divided by 10000, the quotient is 1 and the remainder is 2011. The quotient and the remainder when n is divided by 2011 are respectively.

Answer 1

`Remainder = 1956`

`Quotient = 5`

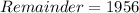
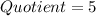
Explanation:

Given

`Dividend = n`

`Divisor = 10000`

`Quotient = 1`

`Remainder = 2011`

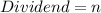
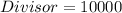
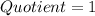
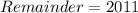
Required

Determine the quotient and remainder when the divisor is 2011

First, we need to determine the value of n.

The relationship between the given parameters is:

`Dividend = Quotient * Divisor + Remainder`

Substitute values in the above:

`n = 1 * 10000 + 2011`

`n = 10000 + 2011`

`n =12011`

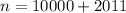
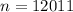
So, we divide 12011 by 2011 and record the quotient and divisor:

`(12011)/(2011) =`

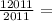
The integer division of 12011 and 2011 is 5.

The remainder is calculated using:

`Dividend = Quotient * Divisor + Remainder`

Where

`Dividend = 12011`

`Quotient = 5`

`Divisor = 2011`

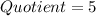
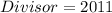
So, Remainder =:

`Remainder = 12011 - 2011* 5`

`Remainder = 12011 - 10055`

`Remainder = 1956`

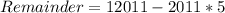
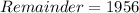
Hence:

`(12011)/(2011) = 5\ Remainder\ 1956`