Question

A parallelogram has an area of 153
cm and height of 17 cm what is the length of the base​

Answer 1

9 cm

Explanation:

Formula :

Area (parallelogram) = Base × Height

=========================================================

Given :

⇒ Area = 153 cm²

⇒ Height = 17 cm

===========================================================

Solving :

⇒ 153 cm² = 17 cm × Base

⇒ Base = 153/17

⇒ Base = 9 cm

Answer 2

Base = 9 cm

Explanation:

Given dimensions:

• Area of the parallelogram: 153 cm^2 ( It should be cm^2 not cm )
• Height of the parallelogram: 17 cm

To Find:

• The base

Solution:

This question is a bit tricky.Usually,the height and the base are given. We'll use the formula of area of parallelogram , then substitute/plug the values of height and area of parallelogram, with the exception of base as it's not given.

So recall the formulae of area of parallelogram:

`\rm \: Area_((Parallelogram)) = b \cdot{h}`

• Here,b = base
• h = height

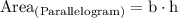
Plug/substitute the values of area of parallelogram & height:

`\rm \: 153 \: cm ^2 =b \cdot17 \: cm`

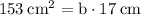
We created an equation.Solve it now.

• Transpose 17 cm to the LHS:

`\implies \rm \cfrac{153 \: cm^2}{17 cm} = base`

• Cancel 153/17 by 17:

`\implies \rm9 \; cm = \: base`

`\implies \rm \: Base = 9\; cm`

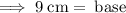
Yes! We're done!

Hence,the base is 9 cm of the parallelogram.

`\rule{225pt}{2pt}`