Question

An eagle is flying horizontally 16.4 meters above a lake at a speed of 9.3 m/s, carrying a small pumpkin in its talons. The pumpkin slips free. How far horizontally will the pumpkin travel after it slips from the eagle until it hits the ground?

Answer 1

The horizontal distance the pumpkin will travel after it slips from the eagle is 17.02 m

Step-by-step explanation:

Given;

height above the ground, h = 16.4 m

speed of the eagle, v = 9.3 m/s

The time it will take the pumpkin to fall at the given height is calculated as;

`t = \sqrt{(2h)/(g) }\\\\t = \sqrt{(2*16.4)/(9.8) }\\\\t = 1.83 \ s`

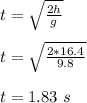
The horizontal distance traveled at this time is given by;

x = vt

x = (9.3)(1.83)

x = 17.02 m

Therefore, the horizontal distance the pumpkin will travel after it slips from the eagle is 17.02 m