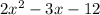Answer:
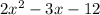
Explanation:
To solve composite functions, figure out which function is on the "inside" and the outside":
f(x) is the inside and g(x) is the outside
Now plug the value of g(x) into the x values of f(x):
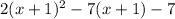
Then distribute following the steps of PEMDAS:
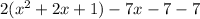
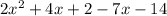
Then combine like terms: