Question

How do you factor f(x) = x^3 – 3x^2 – 10x + 24 ?

Answer 1

f(x) = x³ – 3x² – 10x + 24 = (x + 3)(x – 2)(x – 4)

Explanation:

I would use the Horner method.

f(x) = x³ – 3x² – 10x + 24

f(2) = 2³ - 3·2² - 10·2 +24 = 0 ⇒ x=2 is the root of function

So:

| 1 | -3 | -10 | 24 |

2 | 1 | -1 | -12 | 0 |

therefore:

f(x) = x³ – 3x² – 10x + 24 = (x – 2)(x² – x – 12)

For x² – x – 12:

`x=(1\pm√((-1)^2-4\cdot1\cdot(-12)))/(2\cdot1)=(1\pm√(1+48))/(2)=(1\pm7)/(2)\\\\x_1=(1+7)/(2)=4\ ,\qquad x_2=(1-7)/(2)=-3`

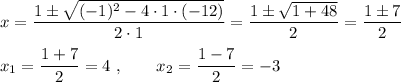
It means:

f(x) = x³ – 3x² – 10x + 24 = (x – 2)(x – 4)(x + 3)