Answer:
16 times
Explanation:

If only the radius is changed, the change in volume will be proportionate to the multiplicative factor squared.
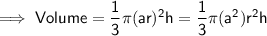
Therefore, if the cone is quadrupled (multiplied by 4), the volume of the larger cone will be 4² times greater than the volume of the smaller cone, so 16 times greater than the smaller cone.
Proof
Given:
Substituting the given values into the formula:
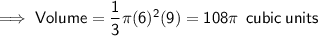
If the radius is quadrupled:
- radius = 6 × 4 = 24
- height = 9
Substituting the new given values into the formula:
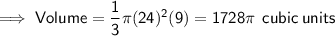
To find the number of times greater the volume of the large cone is than the volume of the smaller cone, divide their volumes:

So the volume of the larger cone is 16 times greater than the volume of the smaller cone.